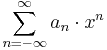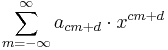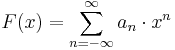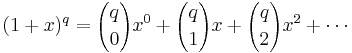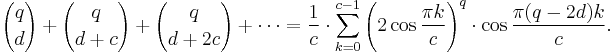Series multisection
In mathematics, a multisection of a power series is a new power series composed of equally-spaced terms extracted unaltered from the original. Formally, if one is given
then a multisection is a power series of the form
where c, d are integers, with 0 ≤ d < c.
Multisection of analytic functions
A multisection of the series of an analytic function
has a closed-form expression in terms of the function 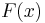 :
:
where 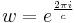 is a primitive c-th root of unity.
is a primitive c-th root of unity.
Example
Multisection of a binomial
at x = 1 gives the following identity for the sum of binomial coefficients with step c:
References
- Weisstein, Eric W., "Series Multisection" from MathWorld.
- Somos, M. A Multisection of q-Series, 2006.
- John Riordan (1968). Combinatorial identities. New York: John Wiley and Sons.